

#REFLECTION ON Y AXIS FUNCTION HOW TO#
If k<0, it's also reflected (or 'flipped') across the x-axis. How to reflect over y axis function - Math Methods. Since h(-1) = -(-1) + 2 = 3, our function demonstrates these translations.Īlthough most problems arise when transforming functions horizontally, order does matter when transforming vertically as well. Scaling & reflecting absolute value functions: equation Google Classroom About Transcript The graph of ykx is the graph of yx scaled by a factor of k. Since our original function was f(x) = x, our new function should be f(-x+2) = -x+2. A vertical reflection reflects a graph vertically across the. Since h(-5) = -(-5)-2 = 3, our function demonstrates these translations.Ī function reflected about the y-axis and then shifted horizontally: Another transformation that can be applied to a function is a reflection over the x or y-axis. Since our original function was f(x) = x, our new function should be f(-x-2) = -x-2. Let's use the function f(x) = x and take the point (3,3) on that function. To understand more clearly, we can take a point on a function as an example. Reflected about the y-axis: g(-x) = f(-x-2) When given the shape graphed on the x y -plane, switch the x and y coordinates to find the resulting image. This process applies even for functions meaning, to reflect a function over y x, switch the input and output values.
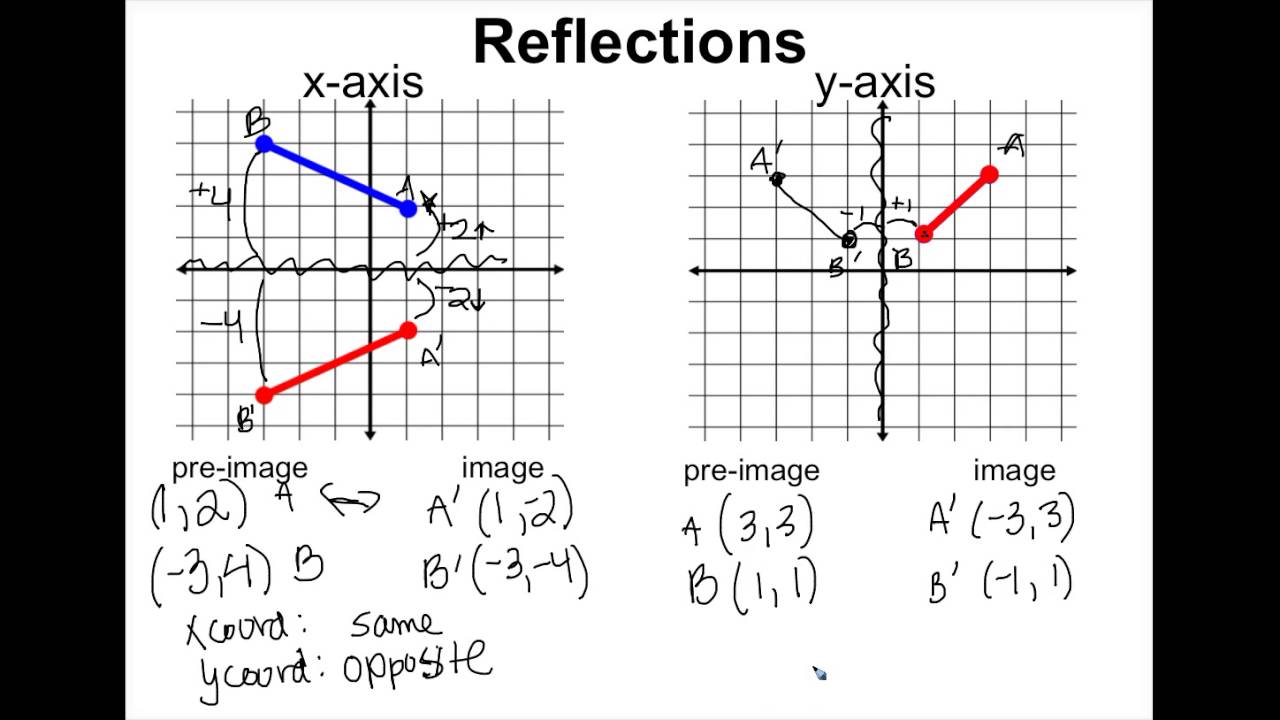
The function retains its basic shape however. To reflect a point or object over the line y x, switch the values of x to y and values of y to x. I demonstrate this on the trigonometric graphs of sine, cosine. If the negative sign belongs to the x, then the graph will flip about the y-axis.A translation of a function horizontally two units to the right and then reflected about the y-axis: A reflection is a transformation of the graph of a function over the x-axis or the y-axis (or both). In this video you are shown how the function yf (-x) transforms the graph of yf (x) as a reflection across the y-axis. If the negative sign belongs to the y, then the graph will flip about the x-axis. Graph functions using compressions and stretches. Determine whether a function is even, odd, or neither from its graph. Graph functions using reflections about the x -axis and the y -axis.

Remember Reflections: They appear like opposites Highlights Learning Objectives In this section, you will: Graph functions using vertical and horizontal shifts. Example: y = | –x| will flip the function about the y-axis If the negative sign belongs to the x-value the graph will reflect about the y-axis. In this example, flipping the original function across the y-axis is identical to the original graph (so it looks like nothing happened). 4 Answers Sorted by: 13 y Af(B(x + C B)) + D y A f ( B ( x + C B)) + D Can be thought of taking f(x) y f ( x) y and performing the following substitution.
Example: y = –|x| will flip the function about the x-axis If the negative sign belongs to the y-value the graph will reflect about the x-axis.ĭo you see how the negative sign is on the inside of the function… affecting the x-value of the function? When you apply a negative to each x-coordinate of each point (-x,y), the graph flips across the y-axis. There are different types of transformations and their graphs, one of which is a math reflection across the y-axis. Question: What does a negative do to a graph? Answer: Multiplying a function by a negative sign creates a reflection: y = –f(x) or y = f( –x)įLIPS FUNCTIONS ABOUT THE X-AXIS y = –f(x)ĭo you see how the negative sign is on the outside of the function… affecting the y-value of the function? When you apply a negative to each y-coordinate of each point (x,-y), the graph flips across the x-axis.


 0 kommentar(er)
0 kommentar(er)
